Meet You at the Intersection: Solving a System of Linear Equations
Experiment #27 from Real-World Math with Vernier
- Education Level
- High School
Introduction
Many times, the solution to a real-life problem involves solving more than one mathematical equation at the same time. The simplest situation of this type involves a pair of equations with two unknown quantities called a linear system. Graphically, the solution to this kind of system represents the point where the graphs of these two lines intersect.
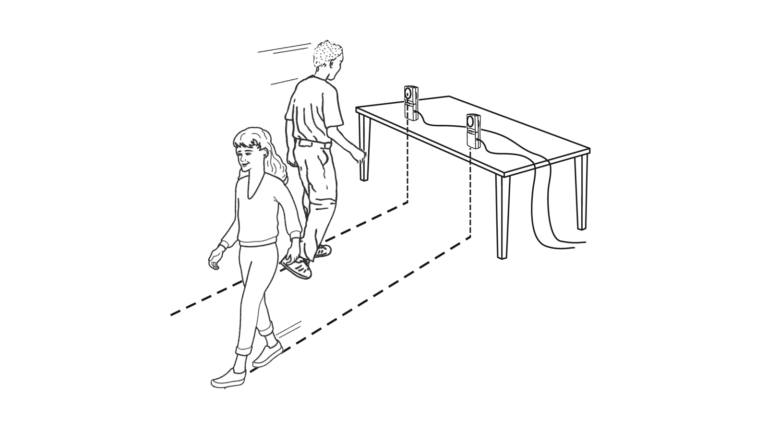
For example, imagine that a person is running to catch up to his friend who is walking ahead of him. These motions can be modeled graphically by plotting distance versus time. The motion graphs are linear if each person is moving at a constant speed. If the plots are made on the same set of axes, the point where the two lines cross represents the physical location where the two friends pass each other.
It is possible to model situations of this type in the classroom using two Motion Detectors. In this activity you will collect and analyze motion data in order to determine the solution to a linear system of equations.
Objectives
- Simultaneously record motion data for two walkers.
- Graph both motions on a common axis and find their intersection.
- Find linear equations to model the motions.
- Solve the system of two linear equations to determine the intersection.
- Compare the algebraic solution to the graphical solution.
Sensors and Equipment
This experiment features the following sensors and equipment. Additional equipment may be required.
Ready to Experiment?
Ask an Expert
Get answers to your questions about how to teach this experiment with our support team.
- Call toll-free: 888-837-6437
- Chat with Us
- Email support@vernier.com
Purchase the Lab Book
This experiment is #27 of Real-World Math with Vernier. The experiment in the book includes student instructions as well as instructor information for set up, helpful hints, and sample graphs and data.




