Experiencing Bluetooth® issues with Go Direct® sensors on Chromebooks? Get help here.
Menu

  • Experiment
  • Swinging Ellipses – Plotting an Ellipse

Experiments​

Swinging Ellipses – Plotting an Ellipse

Experiment #20 from Real-World Math with Vernier

Education Level
High School

Introduction

Any ellipse centered at the origin can be expressed in the form

\frac{{{x^2}}} {{{a^2}}} + \frac{{{y^2}}} {{{b^2}}} = 1

where ± a and ± b represent the x– and y-intercepts of the ellipse.
To graph an ellipse on a calculator, the expression above must first be solved for y to obtain

y = \pm b\sqrt {1 - \frac{{{x^2}}} {{{a^2}}}}

This equation is entered into the calculator in two parts, one expression for the positive part (upper half of the ellipse) and one for the negative part (lower half of the ellipse).

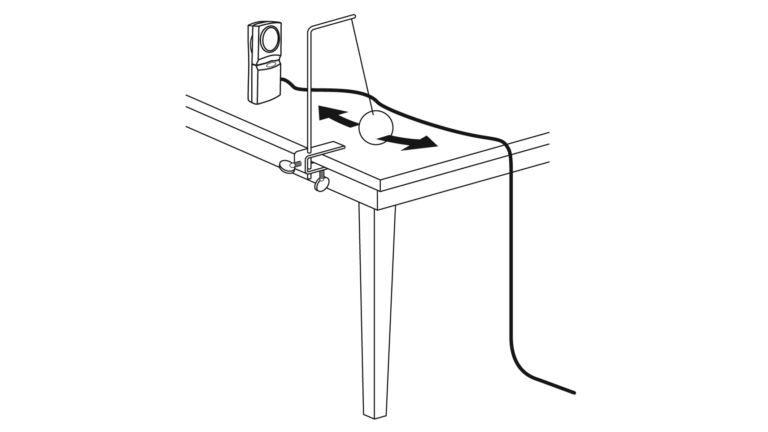
In this activity you will use the Motion Detector to record the position and velocity of a swinging pendulum. You will find that the plot of velocity versus position is elliptical, and that you can model it with the standard equation of an ellipse.

Objectives

  • Record position and velocity versus time data for a swinging pendulum.
  • Plot data as a velocity versus position phase plot.
  • Determine an ellipse that fits the phase plot.

Sensors and Equipment

This experiment features the following sensors and equipment. Additional equipment may be required.

Option 1

Motion Detector

Option 2

CBR 2

Ready to Experiment?

Ask an Expert

Get answers to your questions about how to teach this experiment with our support team.

Purchase the Lab Book

This experiment is #20 of Real-World Math with Vernier. The experiment in the book includes student instructions as well as instructor information for set up, helpful hints, and sample graphs and data.

Learn More

SAVE/SHARE YOUR CART